I just can’t seem to stay away from dialetheism.
*edit*
As you can see the site is experiencing a few technical difficulties, so I’ve reverted to a barebones theme until I can fix the old one. Hang tight.
… almost back to normal now.
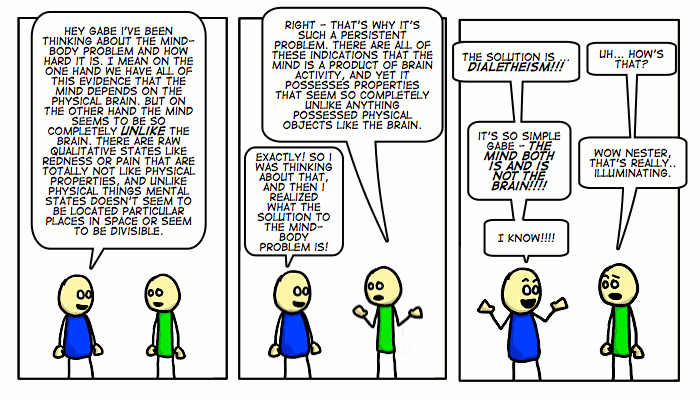
I just can’t seem to stay away from dialetheism.
*edit*
As you can see the site is experiencing a few technical difficulties, so I’ve reverted to a barebones theme until I can fix the old one. Hang tight.
… almost back to normal now.
Dialetheism is annoying. :p
But it solves every problem in philosophy so beautifully!
diathelism. The quantum mechanics of philosophy. 😛
But if Dialetheism solves every problem in philosophy then there is nothing left to talk/discuss/argue about! And thats boring!
Evidently I like talking. A lot.
Has Nester been gaining weight?
We could say that it solves too much. It doesn’t really solve anything. Sort of like invoking God as an explanation for everything.
Emil: That is true! If people 10000 years ago (or however long really) had decided to think about about thunder and lightning in a clear logical manner instead of thinking that some old guy with a beard had decided it was titan smiting time! We could be even more advanced now than we are. We must be wary of Dialetheism in the same way, it works but it doesn’t!
@Emil: Dialetheism is even more powerful than the God explanation, for it can explain some the theistic puzzles as well. For example, can God create a rock so heavy that he can’t lift it? The answer is both yes and no! (so ultimately yes, I agree with you that it isn’t really much of an explanation at all)
@Canuovea: It is still sort of fun explaining how dialetheism “solves” everything.
@Mark: I think his proportions are about the same as they have been for a while.
Ugh. I hate and not-hate and love and not-love dialetheism! 🙁
Though I suppose it would make a very good shroom trip to think about things being true and false at the same time.
By the way, do they (i.e. dialetheists) think everything is true and false, or just some limited amount of things? If limited, then how do they avoid the notorious principle of explosion? Discard some logical inferences so that that argument doesn’t work?
My understanding is that dialetheists generally (certainly Graham Priest) think there is only a very limited set of true contradictions.
As for explosion, Ben has a good take on this – in short, explosion proofs rely on disjunctive syllogism, which is not a valid rule of inference if dialetheism is true.
Uh. Clever.
Here’s another solution to the various paradoxes: Ignore them. They are not important to our general goal of having logic. Things can still be mostly useful even though they are false, think about Newton’s physics.
Emil,
If what you mean by is that we can ignore the paradoxes because orthodox logic can be instrumentally useful even if the paradoxes show it to be strictly speaking false, and this is in some analogous to the way that even within an Einstenian/Quantum framework, Newtonian physics is still instrumentally useful because there’s a certain domain (stuff that’s larger than subatomic particles, not traveling anywhere near the speed of light, etc.) for which Newtonian physics provides an approximately true (although even there, certainly assuming the Coopenhagen Interpretation of quantum mechanics, not anything like 100% true) description, then you’re saying almost exactly what dialetheists like Graham Priest say, although of course they’re curious enough about the question of whether classical logic actually gets things right or is *just* instrumentally useful that they don’t want to *ignore* the paradoxes.
True contradictions would obviously be counter-examples to a few classical rules like Disjunctive Syllogism and Reductio Ad Absurdum, so Priest says these rules aren’t strictly speaking valid (since these rules aren’t *universally* truth-preserving, since he thinks there are some true contradictions), but he also talks about something called the “classical re-capture.” The idea is this. The strictly speaking correct logic is a paraconsistent one that lacks these rules and as such is inferentially weaker than classical logic, but he admits that we do reason with those classical rules all the time without getting into trouble. E.g., you know that the only places Linda could be are at home or at the grocery store, you see that she’s not at home and conclude that she must at the grocery store, and lo, she is.
Priest says that the reason this instance of DS is a rational inference is that classical rules like DS and RAA which rely on the assumption tha there are not true contradictions, although they aren’t deductively valid, still have probabilistic force. The statistical frequency of true contradictions very low–think self-referential sentences, some set-theoretic stuff, arithmetic Gödel sentences, exact moments of change, conflicts of duties in the law or other multi-principle normative systems, and, for Priest, that’s about it–so the epistemic probability of any particular contradiction being true, especially in a domain (like the observable world) where none so far have been discovered, is correspondingly extremely low, and we get to probabilistically re-capture those classical rules that have to be thrown out when reasoning about the “transconsistent.”
(Obviously, I’m not endorsing *any* of this–I have a lot of problems with this–but it is what Priest says.)
I haven’t read any book on the topic but I’ve read a bit on your blog. (Awesome name, by the way. I would use that if you didn’t!) But I think such an approach as you describe above may work, i.e. there is some probability that it is true. Though I’m not convinced that any paradox cannot be resolved within classical logic. It seems one can just dispose of some set of self-referential propositions and the problem disappears. Again, there may be other paradoxes that exist because of different thing, I don’t know.
What do you think are the problems with his (i.e. Priest’s) approach?
“Though I’m not convinced that any paradox cannot be resolved within classical logic. It seems one can just dispose of some set of self-referential propositions and the problem disappears.”
That’s what Bertrand Russel thought. He spent years and years working on the Principia Mathematica, attempting to eliminate the paradoxes from math (and to a lesser extent from logic). Then Goedel came along… He proved in precise terms that any logical system strong enough to reason about the natural numbers was also inherently paradoxical.
In short, you can’t devise a formal system that can both express all truth and NOT contain contradictions.
-Wm
http://xkcd.com/468/
But on a more serious note, you say:
“I’m not convinced that any paradox cannot be resolved within classical logic. It seems one can just dispose of some set of self-referential propositions and the problem disappears.”
That sounds uncomfortably close to “if we ignore the problem, the problem disappears” to me. How exactly do we “dispose” of the problematic sentences? If they express propositions, as you think, and those propositions aren’t true or false (since either would would generate a contradiction), then we’ve reasoned our way out of the framework of classical logic and into some kind of three-valued framework. (Of course, like Priest with his “accept the contradictions and move on” approach, one could attempt a classical re-capture for reasoning about domains in which no truth-value gaps have been discovered, but that’s not quite the same as staying within the framework of classical logic.) If you’re going to say that they don’t express propositions, or are meaningless or whatever, then you both need to have something to say about revenge paradoxes like, for example….
“This sentence is either false or meaningless.”
….and you face the challenge of explaining *why* they’re meaningless. After all, there are clearly meaningful sentences, like (1) and (2) in the series below….
(1) Sentence (2) is false.
(2) Sentence (3) is true.
(3) Hitler won World War II.
….that aren’t about any subject above and beyond the truth-value of a sentence. There are also self-referential sentences, like (5) and (6)…..
(5) This sentence has seven words in it.
(6) This sentence has twenty words in it.
….that are clearly meaningful, and to which we have no problem assigning one or the other of the classical truth-values. Why, then, should we say that a sentence like the Liar that does both of these things isn’t meaningful? And even if we could come up with so since we have Liar-like paradoxes, like Yablo’s series of sentences that each assert of all the ones starting with the next one that they are false–that don’t even involve self-reference per se.
So….disposing….at least disposing in a remotely principled, persuasive way….is tricky.
I’ll think about it and write some thoughts down for you, Ben, and others to read. 🙂
–
Chaospet, how about doing some jokes about the ontological argument? Suppose Nester argued that the ontological argument is sound, and the other guy responded with the perfect island objection, then Nester could concede that with an “awesome” remark, and say that he wants to go there, or something. Worth thinking about it, I think.
[…] heard of it before skholiast’s post, was in two of Ryan Lake’s Chaospet comics that made fun of it. Lake’s comics note apparent problems with dialetheism: if nothing is bad about […]
Dialetheism is line a cheap bandade for a deep paper cut, yah it is accessible, easy to apply and does the trick, but it just isn’t as satisfying and effective as the cloth ones with Spiderman printed on them.